4 things to know about the Fisher formula
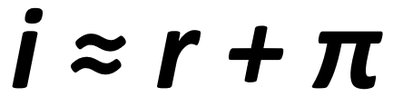
10/29/2021 Jacob Reed
1. What is the Fisher formula?
The Fisher formula shows the relationship between the nominal interest rate, the real interest rate, and the inflation rate.
The precise formula is (1 + nominal interest rate) = (1 + real interest rate) x (1 + inflation rate). Since this formula can be difficult to calculate, a more commonly used formula is i ≈ r +π where i is the nominal interest rate, r is the real interest rate and π is the inflation rate. This second formula is an easy to use approximation that will work great when practicing for the AP Macroeconomics Exam.
2. How does the Fisher formula apply to real and nominal interest rates?
One of the important concepts found on the AP Macroeconomics Exam is the idea that unexpected inflation benefits debtors and hurts creditors. When a bank makes a loan, they will charge a nominal interest rate (i) based on an expected real interest rate (re) and an expected inflation rate (πe). The bank will use the Fisher formula i ≈ re + πe to determine the nominal interest rate to charge.
If the loan is a fixed interest loan, meaning the nominal interest rate does not change with the inflation rate, changes in the actual inflation rate will change the real interest rate paid to the bank.
Example: A college student takes out a fixed interest loan to buy textbooks when the expected inflation rate is 4% and the bank expects to be paid a real interest rate of 3%. The bank will charge a nominal interest rate of 7% (7%≈4%+3%). If the actual rate of inflation ends up being 6%, the real interest rate paid by the college student won’t be the 3% the bank expected but will be just 1% instead (7%≈6%+1%).
One big implication of the fisher formula is that higher than expected rates of inflation will benefit borrowers. That is because borrowers will pay lower real interest rates and pay back their loans with fewer real dollars (or dollars that are less valuable). On the flip side, lenders (banks) will be hurt by higher than expected inflation, as they will be paid lower real interest rates and earn fewer real dollars for their loans.
3. How does the Fisher effect apply to nominal and real GDP?
The Fisher formula can apply to other real vs nominal rates of change too. Differences between nominal and real GDP growth rates can be explained by changes in the price level. The formula is %∆ Nominal GDP ≈ %∆ Real GDP + Inflation Rate.
Example: If real GDP increases by 10% while prices rise by 5%, nominal GDP will increase by approximately 15%
4. How does the Fisher effect apply to nominal and real wage growth?
Just as the Fisher formula can apply to GDP it can apply to real vs nominal wage growth as well. The formula for this relationship %∆ Nominal wage ≈ %∆ Real wage + Inflation Rate.
Example: If Joanne gets a 3% nominal wage increase but her real wage decreases by 2%, then there was approximately 5% inflation (3% ≈ -2% + 5%)
Note: You can use this formula in multiple arrangements to find any of the 3 values.
i ≈ r + π r ≈ i – π π ≈ i – r
Up Next:
Review Game: Fisher Formula practice game
Content Review Page: GDP Deflator and CPI
Other recommended resource: jodiecongirl
